Concept of Critical Value with the help of Different Techniques to find it

48 Comments / Statistics / By / chi-square critical value, chi-square critical values, concept of critical values, critical values, define the concept of critical value, explain the concept of critical value, F-critical values, for F-critical value, for T-critical value, for Z-critical value, hypothesis testing, mitacademys.com, null hypothesis, statistical hypothesis, statistical hypothesis testing, T-critical values, test to determine critical values, two tailed test, types of hypothesis testing, what is the critical value?, what is the critical values, z test in statistics, Z-critical values

A critical value is the test statistic’s value that establishes a confidence interval’s upper and lower boundaries. It explains how far away from the distribution’s mean you must be to account for a specific percentage of the overall variation in the data. For instance, 90%, 95%, and 99%.

Your critical value will be the same in both scenarios if you are creating a 90% confidence interval and utilizing a p = 0.1 as a level of significance. In this article, the basic definition of critical value with formula and also further techniques to find it will be discussed with the help of examples.

What is the Critical Value?

The critical values of a statistical test in statistical hypothesis testing are the limits of the test’s acceptance region. The set of test statistic values for which the null hypothesis is not rejected is known as the acceptance zone. There may be one or many critical values, depending on the geometry of the acceptance region.

In mathematical form it is written as:

Critical value = 1 – (α/2)

Here,

 α = 1 – (confidence level / 100)

In order to test the statistical hypothesis, which provides information about the region in the sampling distribution of test statistics, the critical value was an important factor. In hypothesis testing, the critical value and statistical value are compared to determine whether or not the null hypothesis is accepted.

The significance level and test statistic distribution are used to determine any hypothesis’ crucial value. It utilized two tests. Two-tailed and one-tailed hypothesis tests are also used. One-tailed tests have one critical value, as implied by their name, while two-tailed tests have two critical values.

Tests to Determine Critical Values

There are a few important techniques to evaluate the critical values of any specific sample or population. Commonly used techniques to determine the critical values by statisticians are discussed below:

For T-critical Value:

We utilized the T-test for this value, and the T-test Formula shows the value. This method compares the t-score to the crucial value derived from the T-table. If the t-score is smaller, it indicates that the group is similar, and if it is greater, it indicates that the group is different.

T-value can be evaluated by this step shown below:

  • First of all, the alpha level will be calculated.
  • Subtract 1 from the sample size to get the degree of freedom.
  • With the help of a one-tailed T-table, you can evaluate a one-tailed hypothesis and similarly for a two-tailed hypothesis, a two-tailed T-Table will be used.
  • Now to find the T-Critical value check the box of df (Left side column) and α-value (top row) of the table. Then the selected box will be its critical value.

The formula for a sample test

T= (Y-µ) / (σ/√ n)

Where y shows the sample mean

  • µ is used for the population mean
  • σ is used for the standard deviation
  • n is used for sample size

For Z-critical Value:

It calculates the z-test that lies on the normal distribution if the sample size is more than or equal to 30 and the standard deviation is known then z-test will apply.

Z-value can be evaluated by following the steps below:

  • First of all, the alpha level will be calculated.
  • Subtract the α-level from 0.5 for the one-tailed test and 1 from the α-level for two-tailed tests.
  • Now using the Z table z critical value will be calculated.

Formula:

Z=(Y-µ) / (σ/√ n)

Where “σ” is the standard deviation and “n” is the sample size here.

For F-critical Value:

It can be calculated with F-test and it is used for the comparison of two samples and test statistics are obtained using regression analysis.

The steps for F-value are mentioned below:

  • First of all, the alpha level will be calculated.
  • To get the degree of freedom subtract 1 from sample size 1st.
  • Similarly, subtract 1 from 2nd sample size to get the degree of freedom.
  • Using the F-table you can easily determine F-value.

Formula:

F = (K1)2 / (K2)2

Where K1 and K2 are the standard deviations 1st and 2nd samples respectively.

Chi-square Critical Value:

The chi-square test, which compares sample data with population data, is used to determine this number. This test is used to compare two factors and establish their relationships with one another.

The steps for Chi-value are mentioned below:

  • First of all, the alpha level will be calculated.
  • Subtract 1 from the sample size to get the degree of freedom.
  • With the help of a table, the chi-square value can be calculated.

Examples:

In this section, with the help of examples, the topic will be explained.

Example 1:

Let the one-tailed T-test apply to a sample whose size is 7 and α=0. 5, then calculate the T-critical value.  

Solution:

Step1:

Extract the given data

Sample size = n = 7

Subtract 1 from the sample size to get a degree of freedom (df) = 7 – 1 = 6

Step 2:

To Calculate the value with the help of the T-table.

            T (6, 0.5) = ± 0.7176

So, T-critical value = ± 0.7176

Use online tools to cross-check the result of calculated problems. Let us check the result of the above problem.

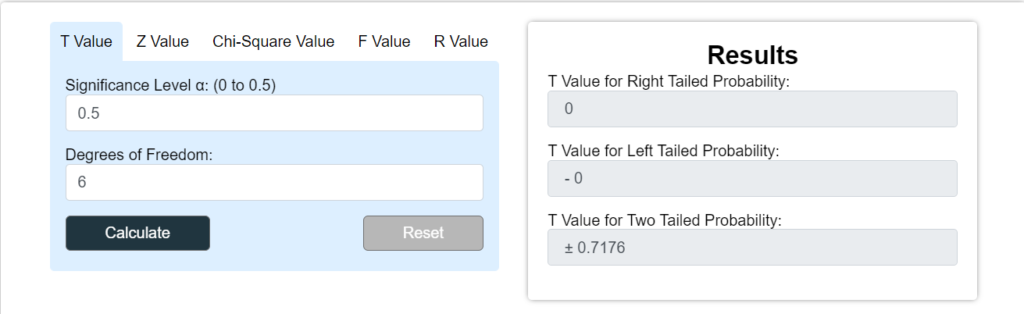
Critical Value
Example source: https://www.criticalvaluecalculator.com/

Example 2:

Let a right-tailed Z-test is applied to the sample whose α-level is 0. 0052. Then find Z-critical Value.

Solution:

Step 1:

Extract the given data

   α -level = 0. 0052

Step 2:

Subtract the α-level from 0.5 for a one-tailed test.

Region indication value = 0.5 -0.0052 = 0. 4948

Step 3:

Calculate the Z-interval with the help of the Z-table.

This value lies between an interval of 2.5 and 0.00

Step 4:

Now Add the intervals to get the Z-value

Z-critical value = 2.5 + 0.00 = 2.5

Z-value = 2.5

Summary:

In this article, the basic definition with its formula and a few important techniques are discussed with their formulas and methods to apply them. Furthermore, with the help of examples topic is explained hope you can easily calculate critical values after a complete understanding of this article.

Rate this post

Related posts

About Libre office. Microsoft office 2016About Microsoft office 2016 About Microsoft Office 2013 Pro Plus. Apache open officeApache Open Office. Variance in Statistics: Definition, Formulas, Properties, and Examples microsoft office 2016About Microsoft office 2016 Hindi About Microsoft Office 2013 Pro Plus Hindi. Trigonometry Class 10th Polynomials Class 10th त्रिकोणमिति कक्षा 10 (The Trigonometry Class 10th) Coordinate Geometry (Analytical Geometry) Class 10th Libre office 5About Libre office In Hindi. Everything About Microsoft Windows 10. Wps officeAbout Wps Office. बहुपद कक्षा 10 (Polynomial Class 10th) Operations on Real Numbers Class 9th tableauAbout Python Tableau Heron’s Formula Class 9th Arithmetic Progression Class 10th Section Formula Class 10th Apache open office hindiApache Open Office Hindi. scipy python libraryAbout Scipy Python Library Quadratic Equation Class 10th mongodbAbout MongoDb Database Sum of First n Terms of an Arithmetic Progression Class 10th Everything About Microsoft Windows 10.Everything About Microsoft Windows 10 Hindi. Triangle and its Properties Class 10th Trigonometric Ratios of Some Specific Angles Class 10th Pair of Linear Equations in Two Variables Class 10th Windows 7About Microsoft Windows 7. कुछ विशिष्ट कोणों के लिए त्रिकोणमितीय अनुपात कक्षा 10 (Trigonometric Ratios of Specific Angles Class 10th) Geometrical (Graphical) Meaning of the Zeroes of a Polynomial Class 10th tailwind cssWhat Is Tailwind Css निर्देशांक ज्यामिति कक्षा 10 (The Coordinate Geometry Class 10th) mysqlAbout mysql बहुपद के शून्यकों का ज्यामितीय (आलेखीय) अर्थ कक्षा 10 [Geometrical (Graphical) Meaning of the Zeroes of the Polynomial Class 10th] Distance Between Two Points in Cartesian Plane and Distance Formula Class 10th nth Term (General Term) of an Arithmetic Progression Class 10th postgresqlAbout Postgresql Windows 10 apps DescriptionMicrosoft Windows 10 Operating System Apps. scala programmingAbout Scala Programming About Raspberry pi board दो चर वाले रैखिक समीकरण युग्म कक्षा 10 (Pair of Linear Equations in Two Variables Class 10th) view.jsAbout Vue.js plsqlAbout Pl/Sql jsonAbout Json json javascript object notation Operators In C Programming english.Operators In C Language. bootstrap cssWhat Is Bootstrap Css bulma cssWhat Is Bulma Css pandasAbout Pandas reactjsAbout ReactJs Framework Windows legacy osMicrosoft Windows Legacy Operating system. विभाजन सूत्र कक्षा 10 (The Section Formula Class 10th) c# programmingAbout C# Programming arduinoAbout Arduino Microcontroller Board Fractions Class 7th Decimal Numbers Class 7th Windows 8.1 appsMicrosoft Windows 8 Apps. Squares and Square Roots Class 8th कार्तीय समतल में दो बिन्दुओं के बीच की दूरी और दूरी सूत्र कक्षा 10 (The Distance Formula Class 10th) xmlAbout Xml sql dbmsAbout Sql – Structure Query Language Operators In C Programming.Operators In C Programming Hindi. Coordinate Geometry Class 9th समान्तर श्रेढ़ी कक्षा 10 (Arithmetic Progressions Class 10th) द्विघात समीकरण कक्षा 10 (Quadratic Equations Class 10th) Perimeter and Area Class 6th python numpyAbout Python Numpy What is linuxLinux, What is Linux, A Complete Overview of Ubuntu Linux. About Microsoft office 365. Data type in c programmingData Type In C Programming. Quadratic Formula (Shridharacharya Sutra) Class 10th Computer Hardware hindiPc assembly and its operation. Integers Class 6th Data type in c programming.Data Type In C Programming Hindi. joomlaAbout Joomla Cms Whole Numbers Class 6th त्रिभुज और इसके गुण कक्षा 10 (The Triangle and its Properties Class 10th) typescript languageAbout Typescript Language Sets Class 11th वर्ग और वर्गमूल कक्षा 8 (Square and Square Root Class 8th) asp.netAbout Asp.net Type of Variable In C Programming.Type of Variable In C Programming. Area of Triangle in Coordinate Geometry Class 10th

Similar Posts

48 thoughts on “Concept of Critical Value with the help of Different Techniques to find it”

  4. Kaiden Wolfe

    This is really interesting, You’re a very skilled blogger. I’ve joined your feed and look forward to seeking more of your magnificent post. Also, I’ve shared your site in my social networks!

    Reply
  8. zebra printer

    You’re so awesome! I don’t believe I have read a single thing like that before. So great to find someone with some original thoughts on this topic. Really.. thank you for starting this up. This website is something that is needed on the internet, someone with a little originality!

    Reply
  13. best movie site

    This is really interesting, You’re a very skilled blogger. I’ve joined your feed and look forward to seeking more of your magnificent post. Also, I’ve shared your site in my social networks!

    Reply
  15. Dominik Li

    Hi there to all, for the reason that I am genuinely keen of reading this website’s post to be updated on a regular basis. It carries pleasant stuff.

    Reply
  16. 9anime

    I’m often to blogging and i really appreciate your content. The article has actually peaks my interest. I’m going to bookmark your web site and maintain checking for brand spanking new information.

    Reply
  17. heres kurdan

    HTML Meta Tag thanks أكبر شركة لانتاج أنابيب البولي ايثيلين و يو بي سي ومستلزماتها للمنتجات الصناعية في عيراق

    Reply
  19. Unknown

    whoah this weblog is great i like studying your articles.
    Stay up the good work! You realize, lots of people are hunting
    around for this information, you can help
    them greatly.

    Reply
  35. Seth Sanders

    This website is a treasure trove of intellectual gems, a testament to the administrator’s passion for knowledge and their commitment to sharing valuable insights. Each article is a masterpiece, meticulously crafted to educate, inspire, and challenge readers. The depth of research, the clarity of thought, and the engaging writing style are a testament to the administrator’s expertise in the subject matter. This website has become my go-to resource for expanding my understanding and staying intellectually stimulated. I am deeply grateful for the administrator’s dedication to delivering exceptional content that nourishes the mind, ignites curiosity, and encourages continuous learning. Their unwavering commitment to providing a platform that enriches the lives of its readers is truly commendable.

    Reply
  36. Unknown

    It’s actually a nice and helpful piece of info. I’m satisfied that you
    just shared this helpful information with us.
    Please stay us informed like this. Thanks for sharing.

    Reply
  38. Aiyana Petty

    Your dedication to delivering high-quality content is evident in every post. I admire your commitment to providing value to your readers. Thank you for the time and effort you invest in your blog.

    Reply
  42. Francesca Weeks

    You’re so awesome! I don’t believe I have read a single thing like that before. So great to find someone with some original thoughts on this topic. Really.. thank you for starting this up. This website is something that is needed on the internet, someone with a little originality!

    Reply
  45. Myles Barr

    I’m often to blogging and i really appreciate your content. The article has actually peaks my interest. I’m going to bookmark your web site and maintain checking for brand spanking new information.

    Reply

Leave a Comment

Your email address will not be published. Required fields are marked *