Coordinate Geometry (Analytical Geometry) Class 10th

Introduction

Coordinate geometry is the topic in which we shall study coordinates of a point, coordinate axes, Cartesian system, plotting of a point, etc. We use coordinates in analytical geometry so it is called Coordinate Geometry.  

Coordinates in Cartesian Plane

In the Cartesian plane, there are two number lines that are perpendicular to each other at a common point. In two number lines, one is a horizontal line which is called the x-axis and the other is a vertical line which is called the y–axis. The common intersection point of both lines is called Origin and it is denoted by O.

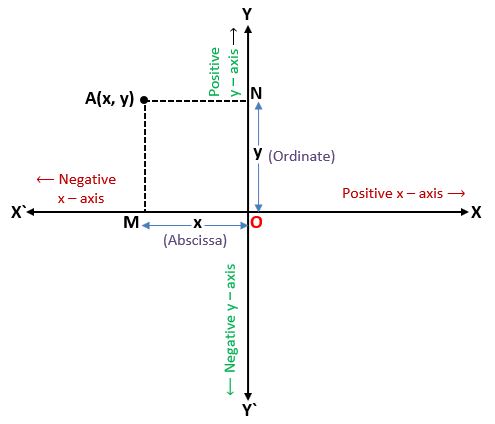
In the above figure, XOX` is a horizontal line which is called the x-axis, and YOY` is a vertical line which is called the y-axis. OX is a positive x-axis and OX` is a negative x-axis and OY is a positive y-axis and OY` is a negative y-axis. Point O is the origin which is the intersection point of XOX` and YOY`.

To find the coordinates of point A, we draw perpendicular on both axes from point A. The perpendicular drawn on the x-axis is AM which is equal to ON, and the perpendicular drawn on the y–axis is AN which is equal to OM.

In the above figure,

AN = OM = x    and     AM = ON = y

Here, for point A, x is the value of x – coordinate (which is also called Abscissa), and y is the value of y – coordinate (which is also called Ordinate). The coordinates of point A are written in the form (x, y). we write x – coordinate first and then y – coordinate in the bracket which is separated by a comma.

Signs of Coordinates in Quadrant

We know that the x-axis and the y–axis intersect each other perpendicularly. In this condition, both the coordinate axes divide the plane into four equal parts which are known as quadrants. The Figure will show you better.

In the figure, the number of Quadrants starts from the side OX(positive x-axis) in an anticlockwise direction. Part XOY is Quadrant I and parts X`OY, X`OY`, and XOY` are Quadrant II, III, and IV respectively.

1. In Quadrant I, both x – coordinate and y – coordinate of a point are positive because of the positive x-axis (OX) and positive y-axis (OY). The Point with a positive x – coordinate and positive y – coordinate will be located in this quadrant.
2. In Quadrant II, the x – coordinate is negative and the y – coordinate is positive because of the negative x-axis (OX`) and positive y-axis (OY). The Point with a negative x – coordinate and positive y – coordinate will be located in this quadrant.
3. In Quadrant III, both x – coordinate and y – coordinate of a point are negative because of the negative x-axis (OX`) and negative y-axis (OY`). The Point with a negative x – coordinate and negative y – coordinate will be located in this quadrant.
4. In Quadrant IV, x – coordinate is positive and y – coordinate is negative because of the positive x-axis (OX) and negative y-axis (OY`). The Point with a positive x – coordinate and negative y – coordinate will be located in this quadrant.

Note – 1) If the x – coordinate and y – coordinate of a point both are zero (x = 0 and y = 0) then that point will be located at the origin. It means the coordinates of Origin are O(0, 0).

2) If x – coordinate is zero (x = 0) and y – coordinate is not zero (y ≠ 0) of a point then that point will be located on the y-axis.

3) If y – coordinate is zero (y = 0) and x – coordinate is not zero (x ≠ 0) of a point then that point will be located on the x-axis.

Sr. No.	Value of Coordinates	Location of Point
1.	x > 0 (Positive) and y > 0 (Positive)	Quadrant I
2.	x < 0 (Negative) and y > 0 (Positive)	Quadrant II
3.	x < 0 (Negative) and y < 0 (Negative)	Quadrant III
4.	x > 0 (Positive) and y < 0 (Negative)	Quadrant IV
5.	x = 0 and y = 0	Origin
6.	x = 0 and y ≠ 0	y-axis
7.	x ≠ 0 and y = 0	x-axis

Distance Between Two Points in Cartesian Plane and Distance Formula

If there are two points situated in the Cartesian plane and we have to find the distance between them, then let’s see how we can find it.

If Two Points are Situated on the Coordinate Axis (Whether on the x-axis or y-axis)

If two points are situated on the x-axis or y-axis then we can easily find the distance between them by taking the Difference. Let there be two points A and B situated on the x-axis and two points C and D situated on the y–axis.

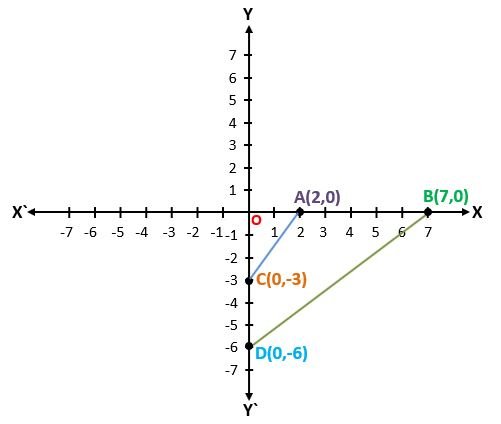
Coordinates of point A = (2,0)      and       Coordinates of point B = (7,0)

Coordinates of point C = (0,-3)     and       Coordinates of point D = (0,-6)

It means the distance of point A from Origin OA = 2 units

and distance of point B from Origin OB = 7 units

Therefore, the distance between points A and B, here OB > OA,    AB = OB – OA = 7 – 2 = 5 units

Similarly, OC = -3 units and OD = -6 units (Here, – sign shows the negative direction)  

So distance between points C and D, here OC > OD, CD = OC – OD = -3 – (-6) = -3 + 6 = 3 units

We can also find the distance between points A & C and points B & D with the help of the Pythagoras theorem (Boudhayana theorem).

At first, we shall join point A to point C and point B to point D. Now we can see there are two right-angled triangles AOC and BOD. By Pythagoras theorem,

In △AOC,  AC² = OA² + OC²

AC = √(2)² + (-3)² = √(4+9) = √13 units

In △BOD,  BD² = OB² + OD²

BD = √(7)² + (-6)² = √(49+36) = √85 units

We saw that if two points are situated on Coordinate axes then we can easily find the distance between them.

If Two Points are not Situated on the Coordinate Axis (Situated in the Quadrant)

Let there be two points P(2,3) and Q(7,5) situated in Quadrant I. To find the distance between points P and Q, we draw perpendicular PR and QS on the x-axis from points P and Q respectively. We also draw perpendicular PT on QS from point P.

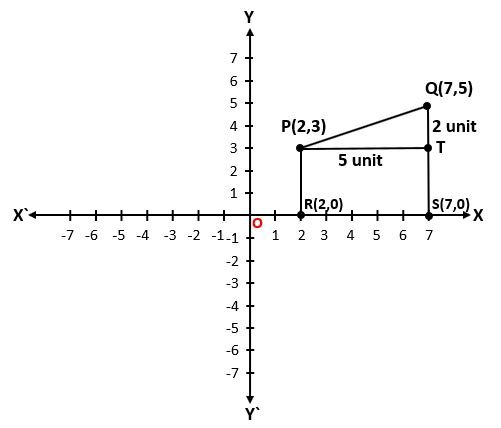
The coordinates of points R and S are (2,0) and (7,0) respectively.

Here, RS = OS – OR = 7 – 2 = 5 units = PT    [∵ RS = PT]

QS = 5 units and PR = 3 units = TS     [∵ PR = TS]

QT = QS – TS = 5 – 3 = 2 units

By Pythagoras theorem, in △PQT,

PQ² = PT² + QT² = (5)² + (2)²

PQ = √(25+4) 

PQ = √29 units

The value of PQ is the distance between two points situated in quadrants.

Distance Formula

Let us consider two points A(x₁,y₁) and B(x₂,y₂) situated in Quadrant I and we have to find the distance AB.

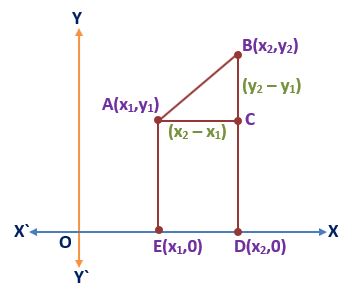
We draw perpendicular AE and BD on the x-axis from points A and B respectively and draw perpendicular AC on BD from point A.

Here,  ED = (x₂ – x₁) units

Since ED = AC, therefore AC = (x₂ – x₁) units    and    BD = y₂ units

AE = CD = y₁ units and BC = BD – CD = (y₂ – y₁) units

In △ABC, by Pythagoras theorem,

AB² = AC² + BC²

AB² = (x₂ – x₁)² + (y₂ – y₁)²

AB = √(x₂ – x₁)² + (y₂ – y₁)²

This expression is called the Distance Formula Because Distance is always positive so, we shall take only the positive value of the square root.

We can also write,

AB = √(Difference of x-coordinates)² + (Difference of y-coordinates)²

Note – 1) Distance of a point A(x,y) from Origin O(0,0) can be written as expression

OA = √(x – 0)² + (y – 0)²

OA = √x² + y²

2) The Distance formula can be also written as

AB = √(x₁ – x₂)² + (y₁ – y₂)²

Because the square of any difference whether negative or positive is always positive.

Let’s Take Some Examples –

Example 1) Find the Distance between points P(2,9) and Q(7,-3).

Solution – Let us compare it with P(x₁,y₁) and Q(x₂,y₂) then

x₁ = 2, y₁ = 9, x₂ = 7, y₂ = -3

By Distance formula,   PQ = √(x₂ – x₁)² + (y₂ – y₁)² 

PQ = √(7 – 2)² + (-3 – 9)²

PQ = √(5)² + (-12)² 

PQ = √(25+144)

PQ = √169 

PQ = 13 units

So, the Distance between points P and Q is 13 units.           Ans.

Example 2) Find the value of x if the Distance between points (1,3) and (x,7) is 5 units.

Solution – Let the given points be A(1,3) and B(x,7).

Distance AB = 5 units, and here, x₁ = 1, y₁ = 3, x₂ = x, y₂ = 7

By Distance formula, AB = √(x₂ – x₁)² + (y₂ – y₁)²

Putting the values,     5 = √(x – 1)² + (7 – 3)²   

5 = √(x² – 2x + 1) + (4)²         [∵ (a – b)² = a² – 2ab + b²]

5 = √(x² – 2x + 1 + 16)

5 = √(x² – 2x + 17) 

Squaring both sides

(5)² = {√(x² – 2x + 17)}²

25 = x² – 2x + 17

x² – 2x + 17 = 25

x² – 2x + 17 – 25 = 0

x² – 2x – 8 = 0

x² – 4x + 2x – 8 = 0     [By factorization method]

x(x – 4) + 2(x – 4) = 0

(x – 4)(x + 2) = 0

(x – 4) = 0      and       (x + 2) = 0

x = 4          and         x = -2

So, the values of x are 4 and -2.             Ans.

More Examples of Distance Formula

Section Formula

In a plane, there are two points whose coordinates are given and are joined by a line and there is also a third point which is situated on the line joining the two points. The third point divides the line into two parts or sections and we have to find the coordinates of the third point.

If we know the ratio of the two sections and the coordinates of the two points then we can easily find out the coordinates of the third point situated on the line joining the two points.

In this there are two conditions, the first being that the third point can be situated in the interior part (on the line joining the two points) which is called the Internal Division and the second is that the third point can be situated in the exterior part (in the left or in the right of both the points) which is called the External Division.

Internal Division of the Distance Between Two Points

Suppose there are two points A(x₁,y₁) and B(x₂,y₂) situated in a plane, and a third point P(x,y) divides the line joining the two points in the ratio m₁ ∶ m₂ internally.

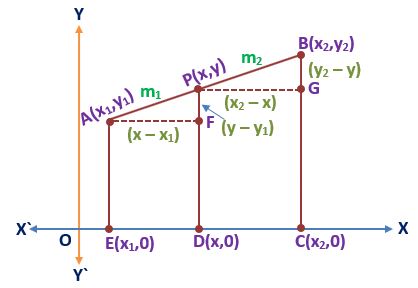
We draw perpendicular AE, BC, and PD on the x–axis from points A, B, and P respectively. We also draw perpendicular AF and PG on PD and BC respectively. From the figure,

OE = x₁,   OD = x,   OC = x₂,   AE = FD = y₁,   PD = GC = y,   BC = y₂

Therefore, AF = ED = x – x₁,    PG = DC = x₂ – x,   

PF = PD – FD = y – y₁,    BG = BC – GC = y₂ – y

And PA/PB = m₁/m₂

In △AFP and △PGB,

∠APF = ∠PBG [Corresponding angles are equal, because PD⊥OX and BC⊥OX, PD∥BC]

∠PAF = ∠BPG [Corresponding angles are equal, because AF⊥PD and PG⊥BC, AF∥PG]

∠AFP = ∠PGB = 90° [AF⊥PD and PG⊥BC]

So, by the AAA similarity criterion, △AFP ∼ △PGB

Therefore, PA/BP = AF/PG = PF/BG  

Putting the values from above

m₁/m₂ = (x – x₁)/(x₂ – x) = (y – y₁)/(y₂ – y) 

Here, m₁/m₂ = (x – x₁)/(x₂ – x)     and   m₁/m₂ = (y – y₁)/(y₂ – y)   

Solving by cross multiply,

m₁(x₂ – x) = m₂(x – x₁)       and      m₁(y₂ – y) = m₂(y – y₁)

m₁x₂ – m₁x = m₂x – m₂x₁   and      m₁y₂ – m₁y = m₂y – m₂y₁

m₁x₂ + m₂x₁ = m₂x+ m₁x   and     m₁y₂ + m₂y₁ = m₂y+ m₁y

m₁x₂ + m₂x₁ = x(m₂ + m₁)      and      m₁y₂ + m₂y₁ = y(m₂ + m₁)

(m₁x₂ + m₂x₁)/(m₂ + m₁) = x       and      (m₁y₂ + m₂y₁)/(m₂ + m₁) = y            

Or       x = (m₁x₂ + m₂x₁)/(m₁ + m₂)         and        y = (m₁y₂ + m₂y₁)/(m₁ + m₂)

The above value of x and y are the required coordinates of point P which divides the line segment AB in the ratio m₁ ∶ m₂ internally.

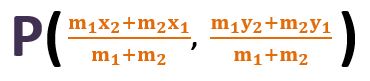
The value of x and y is called the Section Formula for Internal Division.

External Division of the Distance Between Two Points

Suppose there are two points A(x₁,y₁) and B(x₂,y₂) situated in a plane and a third point P(x,y) divides the line joining the two points in the ratio m₁ ∶ m₂ externally.

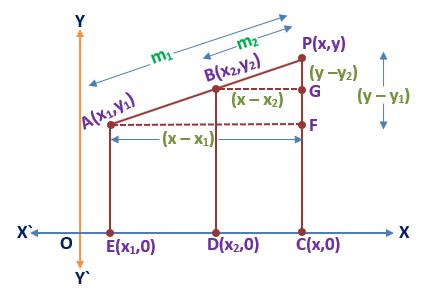
We draw perpendicular AE, BD, and PC on the x–axis from points A, B, and P respectively. We also draw perpendicular AF and BG from points A and B on the PC. From the figure,

OE = x₁,   OD = x₂,   OC = x,   AE = FC = y₁,   BD = GC = y₂,   PC = y

Therefore, AF = EC = x – x₁,    BG = DC = x – x₂,   

PF = PC – FC = y – y₁,    PG = PC – GC = y – y₂

And PA/PB = m₁/m₂

In △AFP and △BGP,

∠APF = ∠BPG [Common angles]

∠PAF = ∠PBG [Corresponding angles are equal, because AF⊥PC and BG⊥PC, AF∥BG]

∠AFP = ∠BGP = 90° [AF⊥PC and BG⊥PC]

So, by the AAA similarity criterion, △AFP ∼ △BGP

Therefore, PA/PB = AF/BG = PF/PG 

Putting the values from above

m₁/m₂ = x – x₁/x – x₂ = y – y₁/y – y₂ 

Here, m₁/m₂ = x – x₁/x – x₂     and      m₁/m₂ = y – y₁/y – y₂   

Solving by cross multiply,

m₁(x – x₂) = m₂(x – x₁)       and      m₁(y – y₂) = m₂(y – y₁)

m₁x – m₁x₂ = m₂x– m₂x₁   and      m₁y – m₁y₂ = m₂y– m₂y₁

m₁x – m₂x = m₁x₂ – m₂x₁    and      m₁y- m₂y = m₁y₂ – m₂y₁

x(m₁ – m₂) = m₁x₂ – m₂x₁   and      y(m₁ – m₂)  = m₁y₂ – m₂y₁

x = (m₁x₂ – m₂x₁)/(m₁ – m₂)         and         y = (m₁y₂ – m₂y₁)/(m₁ – m₂)

The above value of x and y are the required coordinates of point P which divides the line segment AB in the ratio m₁ ∶ m₂ externally.

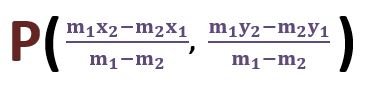
The value of x and y is called the Section Formula for External Division.

Note – 1) In the internal division, If point P is situated in the middle of the line segment AB then it will divide the line segment in the equal ratio as 1 ∶ 1, then coordinates of the mid-point P will be          

x = (1⨯x₂ + 1⨯x₁)/1+1          and           y = (1⨯y₂ + 1⨯y₁)/1+1 

x = (x₂ + x₁)/2 and y = (y₂ + y₁)/2

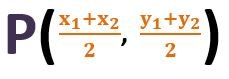
2) In the external division, if m₁ > m₂ then point P will be situated on the right of both points A and B, And if m₂ > m₁ then point P will be situated on the left of both points A and B.

3) The internal division formula can be converted into the External division formula by just replacing the +ve sign with the –ve sign.

4) If point P divides the line segment AB in the ratio which is not known then we can assume the ratio as k ∶ 1 then the coordinates of point P will be [(kx₂ + x₁)/k+1, (ky₂ + y₁)/k+1]

Some Examples –

Example 1) Find the coordinates of the point which divides the line segment joining the points (-2,5) and (3,4) in the ratio 3 ∶ 5 internally.

Solution – Let P(x,y) be the required point that divides the line segment joining the points A(-2,5) and B(3,4). We can understand it by figure.

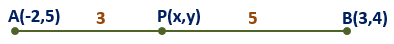
Here, x₁ = -2, y₁ = 5, x₂ = 3, y₂ = 4, m₁ = 3, m₂ = 5

By section formula for internal division,

x = (m₁x₂ + m₂x₁)/(m₁ + m₂)      and      y = (m₁y₂ + m₂y₁)/(m₁ + m₂)

Putting the values,     x = 3⨯3 + 5⨯(-2)/(3 + 5)           and         y = 3⨯4 + 5⨯3/(3 + 5) 

x = 9 + (-10)/8       and         y = 12 + 15/8

x = 9 – 10/8           and         y = 27/8

x = -1/8            and         y = 27/8

So, the required coordinates are (-1/8, 27/8).              Ans.

Example 2) Find the coordinates of the point which externally divides the line segment joining the points (-1,-2) and (-2,4) in the ratio 3 ∶ 2.

Solution – Let the required point be P(x,y).

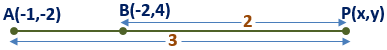
Here, x₁ = -1, y₁ = -2, x₂ = -2, y₂ = 4, m₁ = 3, m₂ = 2

By section formula for external division,

x = (m₁x₂ – m₂x₁)/(m₁ – m₂)      and      y = (m₁y₂ – m₂y₁)/(m₁ – m₂)

Putting the values,     x = 3⨯(-2) – 2⨯(-1)/(3 – 2)         and           y = 3⨯4 – 2⨯(-2)/(3 – 2) 

x = -6 + 2/1          and           y = 12 + 4/1

x = -4         and           y = 16

So, the coordinates of the required point are (-4,16).               Ans.

More Examples of Section Formula

Some Short Type Questions

Q 1) What is Distance Formula?

Ans. – Distance between two points P(x₁,y₁) and Q(x₂,y₂) by Distance Formula

PQ = √(x₂ – x₁)² + (y₂ – y₁)²

Q 2) What is the distance of a point (x,y) from Origin?

Ans. – Distance of a point (x,y) from Origin = √x² + y² 

Q 3) Write section formula for internal and external division.

Ans. – Section formula for internal division ⇒

x = (m₁x₂ + m₂x₁)/(m₁ + m₂)   and   y = (m₁y₂ + m₂y₁)/(m₁ + m₂)

Section formula for external division ⇒

x = (m₁x₂ – m₂x₁)/(m₁ – m₂)     and   y = (m₁y₂ – m₂y₁)/(m₁ – m₂)

Q 4) If a line segment joins the two points (x₁,y₁) and (x₂,y₂) then the coordinates of its mid-point will be? 

Ans. – [(x₁ + x₂)/2, (y₁ + y₂)/2]

Coordinate Geometry Class 10^th in Hindi

More About Coordinate Geometry