Rational Numbers Class 8th

Introduction

In the number system, we have already studied whole numbers, natural numbers, integers, fractions, decimal numbers, etc. All of these types of numbers have different properties. In class 8th, we shall study Rational numbers, their definition and properties, the representation of rational numbers on the number lines, etc.

Definition of Rational Number

A number that can be expressed in the form of p/q, is called a Rational Number. Where p and q are integers and q ≠ 0.

Examples – 1/3, ½, 3/5, -5/2, 7/-8, ¼, etc.

How to Represent Rational Numbers on the Number Line

In the previous class, we learned the representation of natural numbers, whole numbers, integers, fractions, and rational numbers on the number line. There is a method to represent a rational number on the number line. Let us recall the method again with the help of some examples.

Method –

Example 1) Represent rational number ¾ on the number line.

Solution – 1) First of all, we draw the number line with 0 and only positive numbers 1, 2, 3, 4, and 5…. because the given rational number is positive.

2) In the given rational number denominator is 4 so we divide each of the numbers on the number line by 4.

3) In the above number line, we can see the rational number ¾. We mark the rational number ¾ with the help of a circle and arrow.

4) This is the required method to represent a rational number on the number line.

Example 2) Represent rational number -6/5 on the number line.

Solution – 1) First, we draw the number line with 0 and only negative numbers -1, -2, -3, -4, -5…. because the given rational number is negative.

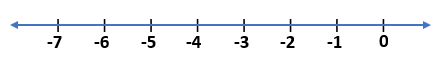
2) We can see the denominator of the given rational number is 5. So, we divide each of the numbers on the number line by 5.

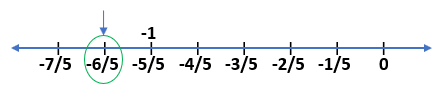
3) Our required rational number is -6/5 and we can see that on the above number line.

4) We mark the rational number -6/5 with the help of a circle and arrow.

5) Thus, we represent the rational number -6/5 on the number line.

Example 3) Represent the rational number 4/9 on the number line.

Solution – 1) First of all, we draw the number line with 0 and only positive numbers 1, 2, 3, 4, 5…. because the given rational number is positive.

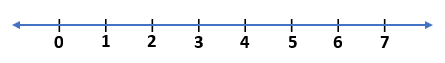
2) In the given rational number denominator is 9 so we divide each of the numbers on the number line by 9.

3) Our required rational number is 4/9 and we can see that on the above number line.

4) We mark the rational number 4/9 with the help of a circle and an arrow.

5) Thus, we represent the rational number 4/9 on the number line.

Properties of the Rational Numbers

Rational Numbers Between Two Rational Numbers

Rational Numbers Class 8th in Hindi

More About Rational Numbers

Related posts

Decimal Expansion of Rational Numbers Class 10th Operations on Real Numbers Class 9th Number System Class 9th Integers Class 6th About Libre office. Irrational Numbers and Irrationality Class 10th Real Numbers Class 8th Fractions Class 7th Tangent and Secant of Circle Class 10th Coordinate Geometry (Analytical Geometry) Class 10th Coordinate Geometry Class 9th Decimal Expansions of Real Numbers Class 9th Apache Open Office. Decimal Numbers Class 7th About Microsoft office 2016 Sets Class 11th Representation of Real Numbers on the Number Line Class 9th Areas Related to Circles Class 10th About Microsoft Office 2013 Pro Plus. Whole Numbers Class 6th Trigonometry Class 10th Angle Subtended by the Arc of a Circle Class 10th Integers Class 7th Polynomials Class 10th Angle Subtended by a Chord of Circle Class 10th Euclid’s Division Lemma Class 10th The Fundamental Theorem of Arithmetic Class 10th परिमेय संख्याएँ कक्षा 8 (Rational Numbers Class 8th) Relations and Functions Class 12th Arithmetic Progression Class 10th Circles Class 10th Powers and Exponents Class 8th Angles of the Alternate Segment of a Circle Class 10th Laws of Exponents for Real Numbers Class 9th Everything About Microsoft Windows 10. Circumference of Circle Class 10th Introduction to Euclid’s Geometry Class 9th परिमेय संख्याओं का दशमलव प्रसार कक्षा 10 (Decimal Expansion of Rational Number Class 10th) Linear Equations in Two Variables Class 9th बहुपद कक्षा 10 (Polynomial Class 10th) Sum of First n Terms of an Arithmetic Progression Class 10th Quadratic Equation Class 10th About Microsoft Office 2013 Pro Plus Hindi. Learn C Programming Pair of Linear Equations in Two Variables Class 10th Section Formula Class 10th HTML Elements nth Term (General Term) of an Arithmetic Progression Class 10th About Microsoft office 2016 Hindi Squares and Square Roots Class 8th Geometrical (Graphical) Meaning of the Zeroes of a Polynomial Class 10th Learn Java Script Programming त्रिकोणमिति कक्षा 10 (The Trigonometry Class 10th) Distance Between Two Points in Cartesian Plane and Distance Formula Class 10th Heron’s Formula Class 9th Triangle and its Properties Class 10th Cyclic Quadrilaterals Class 10th वास्तविक संख्याओं पर संक्रियाएँ कक्षा 9वीं (Operations on Real Numbers Class 9th) About Libre office In Hindi. About Vue.js संख्या रेखा पर वास्तविक संख्याओं का निरूपण कक्षा 9वीं (Representation of Real Numbers on the Number Line Class 9th) Learn Html5 त्रिभुज और इसके गुण कक्षा 10 (The Triangle and its Properties Class 10th) Learn Html संख्या पद्धति कक्षा 9वीं (Number System Class 9th) About mysql About Json json javascript object notation About Xml About Pandas वृत्त की जीवा द्वारा अंतरित कोण कक्षा 10 (Angle Made by a Chord of Circle Class 10th) निर्देशांक ज्यामिति कक्षा 10 (The Coordinate Geometry Class 10th) About MongoDb Database What Is Tailwind Css वृत्त की स्पर्श रेखा और छेदक रेखा कक्षा 10 (Tangent and Secant of Circles Class 10th) Perimeter and Area Class 6th वास्तविक संख्याओं का दशमलव प्रसार कक्षा 9वीं (Decimal Expansion of Real Numbers Class 9th) Everything About Microsoft Windows 10 Hindi. About ReactJs Framework Apache Open Office Hindi. Variance in Statistics: Definition, Formulas, Properties, and Examples एक वृत्त के चाप द्वारा अंतरित कोण कक्षा 10 (Angles Made by the Arc of Circle Class 10th) About Python Tableau Microsoft Windows 10 Operating System Apps. About Scipy Python Library About Wps Office. वृत्तों से संबंधित क्षेत्रफल कक्षा 10 (Areas Related to Circle Class 10th) Linear Equations in One Variable Class 8th What Is Bootstrap Css What Is Bulma Css Area of Triangle in Coordinate Geometry Class 10th समान्तर श्रेढ़ी कक्षा 10 (Arithmetic Progressions Class 10th) About Scala Programming Learn Java Programming NCERT Maths Class 10th Solution Probability Class 10th Microsoft Windows Legacy Operating system. About Raspberry pi board About Microsoft Windows 7. Circles Passing Through Different Points Class 10th About Arduino Microcontroller Board HTML Elements In Hindi Microsoft Windows 8 Apps. Lines and Angles Class 9th एक वृत्त के एकान्तर खंड के कोण कक्षा 10 (Angles of Alternate Segment of Circle Class 10th) वृत्त की परिधि कक्षा 10 (The Circumference of Circle Class 10th) Polynomials Class 9th वास्तविक संख्याएँ कक्षा 8 (Real Number Class 8th) वृत्त और इसके भाग कक्षा 10 (Circle and its Parts Class 10th) द्विघात समीकरण कक्षा 10 (Quadratic Equations Class 10th) How can you Understand the Acids, Bases, and Salts? Surface Areas and Volumes Class 10th Operations on Polynomials Class 10th वास्तविक संख्याओं के लिए घातांक के नियम कक्षा 9 (Laws of Exponents for Real Numbers Class 9th) हीरोन का सूत्र कक्षा 9 (Heron’s Formula Class 9th) The Similarity of Triangles Class 10th About Postgresql Linux, What is Linux, A Complete Overview of Ubuntu Linux. बहुपद के शून्यकों का ज्यामितीय (आलेखीय) अर्थ कक्षा 10 [Geometrical (Graphical) Meaning of the Zeroes of the Polynomial Class 10th] Computer Fundamental. Trigonometric Ratios of Some Specific Angles Class 10th What Is A Search Engine and Their Type? About Pl/Sql समान्तर श्रेढ़ी का n वाँ पद (व्यापक पद) कक्षा 10 (nth Term of Arithmetic Progression Class 10th) What is Nodejs परिमाप और क्षेत्रफल कक्षा 6 (Perimeter and Area for Class 6th) समान्तर श्रेढ़ी के पहले n पदों का योग कक्षा 10 (Sum of n Terms of Arithmetic Progression Class 10th) About Magento Programming यूक्लिड की ज्यामिति का परिचय कक्षा 9 (Introduction to Euclid’s Geometry Class 9th) About C# Programming Relation Between Trigonometric Ratios Class 10th About Python Numpy समुच्चय कक्षा 11 (The Sets Class 11th) Zeroes of the Polynomials Class 10th Mensuration Q & A Quadratic Formula (Shridharacharya Sutra) Class 10th Operators In C Language. About Microsoft Windows 7 Hindi. About Kotlin Programming Some Applications of Trigonometry Class 10th Division Algorithm, Remainder Theorem, and Factor Theorem Class 10th दशमलव संख्याएँ कक्षा 7 (Decimal Number Class 7th) Trigonometric Ratios of Acute Angles Class 10th अपरिमेय संख्या और अपरिमेयता कक्षा 10 (Irrational Number and Irrationality Class 10th) NCERT गणित कक्षा 10 अध्याय 1 वास्तविक संख्याएँ हल What Is Express.js Microsoft Windows 10 Apps Hindi. Input And Output Device. What is Angular Js Pythagoras Theorem (Boudhayana Theorem) Class 10th विभाजन सूत्र कक्षा 10 (The Section Formula Class 10th)