Relation Between Trigonometric Ratios Class 10th

Introduction

We know that the reciprocal of sin ϴ, cos ϴ, and tan ϴ is cosec ϴ, sec ϴ, and cot ϴ respectively. Therefore, some formulae are here which show the Relation Between Trigonometric Ratios.

Derivation of Formula and Explanation

If we assume △PQR, 

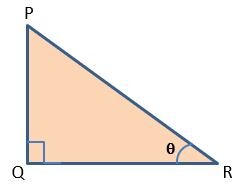
sin ϴ = PQ/PR    and cosec ϴ = PR/PQ

sin ϴ⨯cosec ϴ = PQ/PR⨯PR/PQ

sin ϴ⨯cosec ϴ = 1

Similarly,

1) sin ϴ×cosec ϴ = 1  ⇒      sin ϴ = 1/cosec ϴ and       cosec ϴ = 1/sin ϴ 

2) cos ϴ×sec ϴ = 1   ⇒      cos ϴ = 1/sec ϴ     and     sec ϴ = 1/cos ϴ 

3) tan ϴ×cot ϴ = 1   ⇒    tan ϴ = 1/cot ϴ     and     cot ϴ = 1/ tan ϴ 

4) tan ϴ = sin ϴ/cos ϴ

∵ sin ϴ = PQ/PR         and          cos ϴ = QR/PR

Now, sin ϴ/cos ϴ = PQ/PR ∕ QR/PR = PQ/PR×PR/QR = PQ/QR = Perpendicular/Base = tan ϴ

Similarly,

5) cot ϴ = cos ϴ/sin ϴ

Trigonometric Identities

(1) sin² ϴ + cos² ϴ = 1

∵ sin ϴ = PQ/PR  and  cos ϴ = QR/PR [from the above figure]

LHS       

sin² ϴ + cos² ϴ

(PQ/PR)² + (QR/PR)² 

PQ²/PR² + QR²/PR²

PQ² + QR²/PR²

PR²/PR²  = 1 = RHS           (by Pythagoras theorem PR² = PQ² + QR²)

(2) 1 + tan² ϴ = sec² ϴ

∵ tan ϴ = PQ/QR [from the above figure]

LHS  

1 + tan² ϴ

1 + (PQ/QR)²

1 + PQ²/QR²

QR² + PQ²/QR² 

PR²/QR² = (PR/QR)² = sec² ϴ = RHS   

Alternate Method –

We know that,  sin² ϴ + cos² ϴ = 1

Divide both sides by cos² ϴ

sin² ϴ/cos² ϴ + cos² ϴ/cos² ϴ = 1/cos² ϴ               (∵ tan ϴ = sin ϴ/cos ϴ)

tan² ϴ + 1 = sec² ϴ   

(3) 1 + cot² ϴ = cosec² ϴ

∵ cot ϴ = QR/PQ [from the above figure]

LHS    

1 + cot² ϴ

1 + (QR/PQ)²

1 + QR²/PQ² 

PQ² + QR²/PQ² 

PR²/PQ² = (PR/PQ)² = cosec² ϴ = RHS

Alternate Method –

We know that,  sin² ϴ + cos² ϴ = 1

Divide both sides by sin² ϴ

sin² ϴ/sin² ϴ + cos² ϴ/sin² ϴ = 1/sin² ϴ            (∵ cot ϴ = cos ϴ/sin ϴ)  

1 + cot² ϴ  = cosec² ϴ

Note – 1) (sin ϴ)² = sin² ϴ ≠ sin ϴ²

It means (sin ϴ)² can be written as sin² ϴ but cannot be written as sin ϴ². The same applies to other trigonometric functions.

2) Trigonometric identities can be written as –

sin2 ϴ + cos2 ϴ = 1	1 + tan2 ϴ = sec2 ϴ	1 + cot2 ϴ  = cosec2 ϴ
sin2 ϴ = 1 – cos2 ϴ	sec2 ϴ – tan2 ϴ = 1	cosec2 ϴ – cot2 ϴ = 1
cos2 ϴ = 1 – sin2 ϴ	tan2 ϴ = sec2 ϴ – 1	cot2 ϴ = cosec2 ϴ – 1

Example – If sin ϴ = 5/13 then find all the trigonometric functions using the relation between trigonometric ratios, where ϴ is an acute angle.

Solution – Here, sin ϴ = 5/13 

We know that   sin² ϴ + cos² ϴ = 1

(5/13)² + cos² ϴ = 1

cos² ϴ = 1 – (25/169)

cos ϴ = √(169-25)/169 = √(144/169) = 12/13 

∵ sec ϴ = 1/cos ϴ  

sec ϴ = 1 ∕ 12/13 = 13/12  

∵ cosec ϴ = 1/sin ϴ = 1 ∕ 5/13 = 13/5  

∵ 1 + cot² ϴ = cosec² ϴ

cot² ϴ = cosec² ϴ – 1

cot ϴ = √(13/5)² – 1= √169/25 – 1 = √(169-25)/25 = √(144/25) = 12/5  

∵ tan ϴ = 1/cot ϴ

tan ϴ = 1 ∕ 12/5 = 5/12  

Examples Based on Trigonometric Identities

Example – 1) Prove that tan ϴ + cot ϴ = sec ϴ×cosec ϴ

Solution – tan ϴ + cot ϴ = sec ϴ×cosec ϴ

LHS         tan ϴ + cot ϴ

sin ϴ/cos ϴ + cos ϴ/sin ϴ

(sin ϴ⨯sin ϴ + cos ϴ⨯cos ϴ)/cos ϴ⨯sin ϴ

(sin² ϴ + cos² ϴ)/cos ϴ⨯sin ϴ {∵ sin² ϴ + cos² ϴ = 1}

(1/cos ϴ)⨯sin ϴ = sec ϴ × cosec ϴ = RHS

Example – 2) Prove that (1 + cot² ϴ)(1 + cos ϴ)(1 – cos ϴ) = 1

Solution – (1 + cot² ϴ)(1 + cos ϴ)(1 – cos ϴ) = 1

LHS          (1 + cot² ϴ)(1 + cos ϴ)(1 – cos ϴ) {∵ 1 + cot² ϴ = cosec² ϴ}

(cosec² ϴ)(1 – cos²ϴ)      {(a+b)(a-b) = a² – b²}

cosec² ϴ×sin² ϴ {∵ 1 – cos² ϴ = sin² ϴ}

(1/sin²ϴ)×sin²ϴ               {cosec ϴ = 1/sinϴ}

1 = RHS

Relation Between Trigonometric Ratios Class 10^th in Hindi

More About Relation Between Trigonometric Ratios