Introduction
Before finding the Area of a Triangle in Coordinate Geometry, We know the area of a triangle if its Base and Height are given
Area of triangle = ½⨯Base⨯Height
If all three sides of a triangle are given then the area of the triangle by Heron’s formula
Area of triangle = √s(s-a)(s-b)(s-c)
Where, s = semi-perimeter of the triangle
s = a+b+c/2
a,b,c = three sides of triangle
Derivation of Formula
In coordinate geometry, if coordinates of all three vertices of a triangle are given then we can calculate the area of that triangle. Let us see how it is possible.
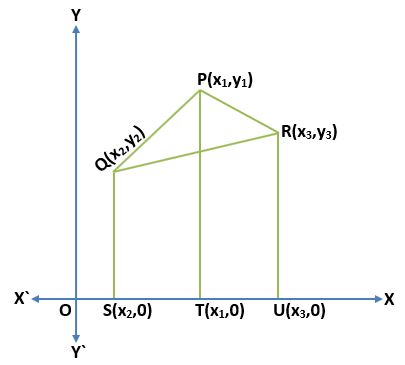
Let PQR be a triangle whose vertices are P(x1,y1), Q(x2,y2), and R(x3,y3). We have drawn perpendiculars QS, PT, and RU from the points Q, P, and R respectively on the x-axis. In the figure, we can see that there are three trapezium PQST, PRUT, and QSUR.
Here, ST = x1 – x2, TU = x3 – x1, SU = x3 – x2
QS = y2, PT = y1, RU = y3
So, we can write,
area of triangle PQR = (area of trapezium PQST + area of trapezium PRUT) – area of trapezium QSUR —————(1)
We know that area of trapezium = ½⨯(sum of parallel sides)⨯(distance between them)
Now area of trapezium PQST = ½⨯(QS+PT)⨯ST
= ½⨯(y2 + y1)⨯(x1 – x2)
= ½(x1y2 – x2y2 + x1y1 – x2y1) ——————–(2)
Area of trapezium PRUT = ½⨯(PT+RU)⨯TU
= ½⨯(y1+y3)⨯(x3 – x1)
= ½(x3y1 – x1y1 + x3y3 – x1y3) ———————(3)
Area of trapezium QSUR = ½⨯(QS+RU)⨯SU
= ½ ⨯(y2+y3)⨯(x3 – x2)
= ½ (x3y2 – x2y2 + x3y3 – x2y3) ———————(4)
From equations (1), (2), (3) and (4)
Area of triangle PQR = ½(x1y2 – x2y2 + x1y1 – x2y1) + ½(x3y1 – x1y1 + x3y3 – x1y3) – ½(x3y2 – x2y2 + x3y3 – x2y3)
= ½[x1y2 – x2y2 + x1y1 – x2y1 + x3y1 – x1y1 + x3y3 – x1y3 – x3y2 + x2y2 – x3y3 + x2y3]
= ½[x1y2 – x2y1 + x3y1 – x1y3 – x3y2 + x2y3]
= ½[x1(y2 – y3) + x2(y3 – y1) + x3(y1 – y2)]
Thus, area of triangle PQR = ½[x1(y2 – y3) + x2(y3 – y1) + x3(y1 – y2)]
Look at Some Examples
Example 1) Find the area of a triangle whose vertices are (-1,1), (6,-4), and (-5,-3).
Solution – Here, the vertices of the triangle are (-1,1), (6,-4), and (-5,-3).
So, x1 = -1, y1 = 1, x2 = 6, y2 = -4, x3 = -5, y3 = -3
By the formula,
area of triangle = ½[x1(y2 – y3) + x2(y3 – y1) + x3(y1 – y2)]
= ½[-1{-4 – (-3)} + 6(-3 – 1) + (-5){1 – (-4)}]
= ½[-1{-4 + 3} + 6(-4) + (-5){1 + 4}]
= ½[-1{-1} + (-24) + (-5){5}]
= ½[1 – 24 – 25] = ½[1 – 49] = ½[- 48] = -24 square units
Since the area is a positive quantity, that can’t be negative. So we shall take positive measures only.
Therefore, the area of the triangle = 24 square units Ans.
Example 2) Find the area of the triangle ABC made by vertices A(2,5), B(7,4), and C(-4,7).
Solution – Here, x1 = 2, y1 = 5, x2 = 7, y2 = 4, x3 = -4, y3 = 7
Now area of triangle ABC = ½[x1(y2 – y3) + x2(y3 – y1) + x3(y1 – y2)]
= ½[2(4 – 7) + 7(7 – 5) + (-4)(5 – 4)]
= ½[2(– 3) + 7(2) + (-4)(1)]
= ½[– 6 + 14 – 4]
= ½[4] = 2 square units
So, the area of triangle ABC = 2 square units. Ans.
Example 3) The vertices of a triangle are (3,2), (0,4), and (-3,6). Find its area.
Solution – Here, x1 = 3, y1 = 2, x2 = 0, y2 = 4, x3 = -3, y3 = 6
Area of triangle = ½[x1(y2 – y3) + x2(y3 – y1) + x3(y1 – y2)]
= ½[3(4 – 6) + 0(6 – 2) + (-3)(2 – 4)]
= ½[3(– 2) + 0 + (-3)(– 2)]
= ½[– 6 + 6] = ½[0] = 0
Here, the area of the triangle is 0 which means the three vertices given in the question do not make a triangle or we can say that the given points are collinear. Ans.
Note – If three points are given and we have to check that they are collinear or not then we can check this with the help of the area of the triangle formula. If the area of a triangle is zero (0) it means the given three points are collinear and if the area of a triangle is not zero(≠0) it means the given three points are non-collinear.
Example 4) Check whether the three points (-1,2), (-2,3) and (-3,4) are collinear or not.
Solution – To check whether three points (-1,2), (-2,3), and (-3,4) are collinear or not we shall find that they form a triangle or not with the help of the area of the triangle formula.
Here, x1 = -1, y1 = 2, x2 = -2, y2 = 3, x3 = -3, y3 = 4
Area of triangle = ½[x1(y2 – y3) + x2(y3 – y1) + x3(y1 – y2)]
= ½[-1(3 – 4) + (-2)(4 – 2) + (-3)(2 – 3)]
= ½[-1(– 1) + (-2)(2) + (-3)( – 1)]
= ½[1 – 4 + 3] = ½[0] = 0
Since the area of the triangle formed by the given three points is 0 so these points are collinear. Ans.
Example 5) Find the value of P if the points (2,-1), (P,-2), and (4,-3) are collinear.
Solution – Since points (2,-1), (P,-2), and (4,-3) are collinear so the area of the triangle formed by these points will be 0.
Here, x1 = 2, y1 = -1, x2 = P, y2 = -2, x3 = 4, y3 = -3
∵ Area of triangle = 0
½[x1(y2 – y3) + x2(y3 – y1) + x3(y1 – y2)] = 0
½[2{-2 – (-3)} + P{-3 – (-1)} + 4{-1 – (-2)}] = 0
½[2{-2 + 3} + P{-3 + 1} + 4{-1 + 2}] = 0
½[2{1} + P{-2} + 4{1}] = 0
½[2 – 2P + 4] = 0
½[6 – 2P] = 0
6 – 2P = 0
6 = 2P
6/2 = P
P = 3
Therefore, the value of P is 3. Ans.
Some Questions Based on it
Q 1) Write the formula to find the area of a triangle if its vertices are given.
Ans. – Area of a triangle = ½[x1(y2 – y3) + x2(y3 – y1) + x3(y1 – y2)]
Q 2) How can we check that the three points are collinear or not with the help of the area of a triangle?
Ans. – If the area of the triangle formed by that three points is zero (0) then we can say that the three points are collinear and if the area of a triangle is not zero(≠0) then the three points are non–collinear.
Q 3) What are collinear and non-collinear points?
Ans. – Collinear Points – If three or more points lie on a straight line, they are called collinear points.

Non-collinear Points – If three or more points do not lie on a straight line, they are called non–collinear points.











































































































































I pay a visit each day some web pages and blogs to read articles or reviews, but this webpage gives quality based articles.