Relationship Between Zeroes and Coefficients of a Quadratic Polynomial Class 10th

Introduction

We use the standard form of a quadratic polynomial to find the Relationship between Zeroes and Coefficients of a Quadratic Polynomial. We know the standard form of a quadratic polynomial is f(x) = ax² + bx + c.

Derivation of Formula

Let α and β be the two zeroes of this polynomial. Then (x – α) and (x – β) will be factors of f(x).

So, for constant k we can write

f(x) = k(x – α) (x – β)

ax² + bx + c = k{x² – (α + β)x + αβ}

ax² + bx + c = kx² – k(α + β)x + kαβ

by comparing,     a = k,   b= – k(α + β),   c = kαβ

∵ b= – k(α + β)       and c = kαβ

α + β = b/-k         and       αβ = c/k

∵ a = k                                   

α + β = b/-a or -b/a     and        αβ = c/a

So, for quadratic polynomial f(x) = ax² + bx + c

sum of zeroes (α + β) = -b/a = -(coefficient of x)/(coefficient of x²)

product of zeroes (αβ) = c/a = (constant term)/(coefficient of x²)

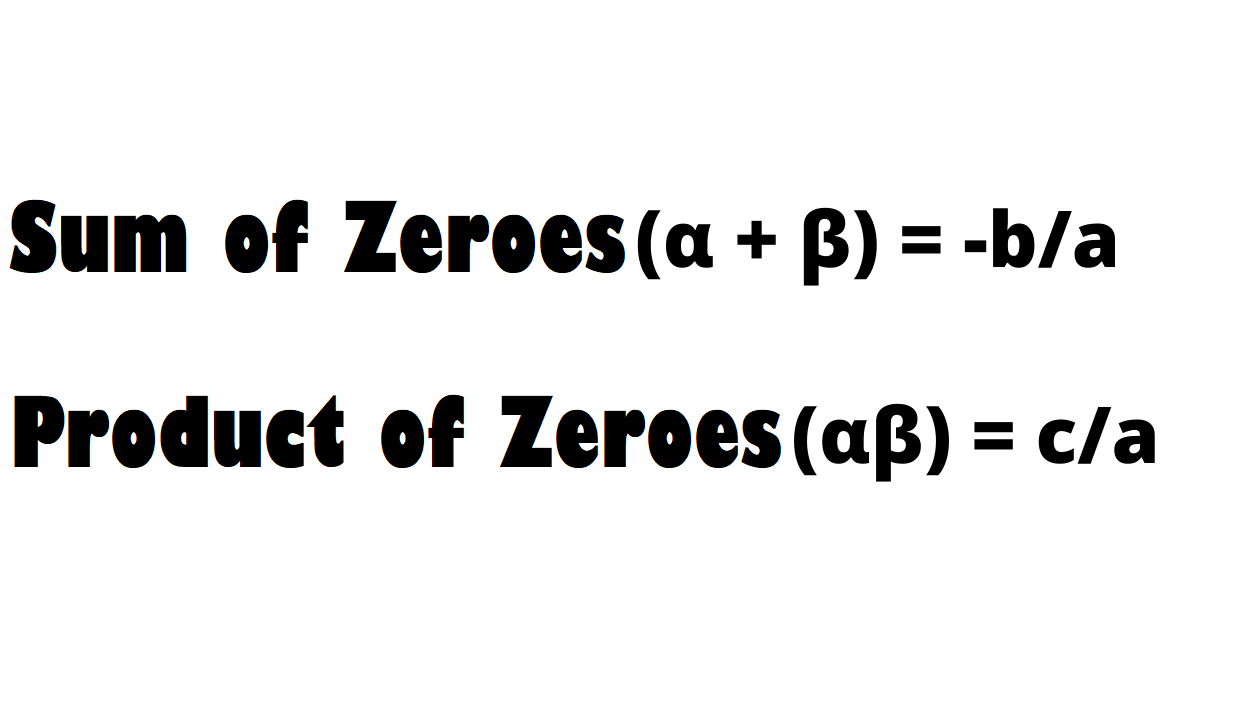
Some Examples

Example – 1) find the zeroes of the quadratic polynomial 3x² + 5x – 2 and verify the relationship between the zeroes and the coefficients.

Solution – let f(x) = 3x² + 5x – 2

Now f(x) = 0

3x² + 5x – 2 = 0

3x² + 6x – x – 2 = 0

3x(x + 2) – 1(x + 2) = 0

(x + 2)(3x – 1) = 0

x + 2 = 0     and     3x – 1 = 0

x = – 2       and         x = ⅓

So, the zeroes of polynomial f(x) are x = – 2 and x = ⅓

Now sum of the zeroes = – 2 + ⅓ = (-6 + 1)/3 = -5/3 = -(coefficient of x)/(coefficient of x²) 

product of zeroes = – 2⨯⅓ = -2/3 = (constant term)/(coefficient of x²)

So, the relationship between the zeroes and the coefficients of the quadratic polynomial is verified.

Example – 2) find a quadratic polynomial, whose sum and product of zeroes are -6 and 5 respectively.

Solution – let α and β be the zeroes of a quadratic polynomial.

For any constant k, the quadratic polynomial will be

k{x² – (α + β)x + αβ}

In question, the sum of zeroes (α + β) = – 6

product of zeroes (αβ) = 5

putting the values,

k{x² – (- 6)x + 5}

k{x² + 6x + 5}       [Where k = Constant]           

So, the required quadratic polynomial is x² + 6x + 5.        Ans.

Example – 3) find all the zeroes of polynomial f(x) = x³ + 13x² + 32x + 20, if it’s one zero is – 2.

Solution – Here, – 2 is zero so the factor will be (x + 2). It is one factor of f(x).

To find other zeroes we will divide polynomial f(x) by factor (x + 2).

From the division algorithm, quotient x2 + 11x + 10 will be its factor because the remainder is 0.

Now          Dividend = Divisor ⨯ Quotient + Remainder

x³ + 13x² + 32x + 20 = (x + 2)⨯(x² + 11x + 10) + 0

x³ + 13x² + 32x + 20 = (x + 2)⨯{ x² + 10x + x + 10}

x³ + 13x² + 32x + 20 = (x + 2)⨯{ x(x + 10) + 1(x + 10)}

x³ + 13x² + 32x + 20 = (x + 2)⨯(x + 10)(x + 1)

To find zeroes, f(x) = 0

(x + 2)(x + 10)(x + 1) = 0

x = -2, x = -10 and x = -1

So, all the zeroes of polynomial f(x) are -2, -10, and -1. Ans.

Relationship Between Zeroes and Coefficients of a Quadratic Polynomial Class 10^th in Hindi

More About Relationship Between Zeroes and Coefficients of a Quadratic Polynomial